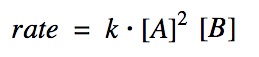Methods for determining order of reaction
This section looks at how concentration affects reaction rate. You will learn how to determine the order of a reaction. Reactions are often categorized into first, second, third order, etc. and this information is useful for showing the mathematical relationship between concentrations and rates.
Rate laws may be written using two different but related perspectives:
- A differential rate law
- An integrated rate law
In this section, we will focus on differential rate laws, integrated rate laws will be discussed in detail in the next page. Before we begin, download a flowchart here that will help you navigate this lesson:
Rate of reaction
The rate of reaction studies how fast a reaction takes place – which is concerned with speed. The study relates the change in concentration (reactant or product) over time elapsed.
[concentration] vs time
Order of reaction
The order of reaction studies how a reaction’s rate is affected by the concentration of each reactant in the reaction. The studies relate to the change in concentration (reactants) to the rate of reaction.
rate vs [concentration]
Differential rate law equation
In calculating order of reaction, we are only interested in the concentration of the reactants and NOT the products.
A very simple reaction A + B → C + D, where A and B are the reactants and C and D are the products, we can find the order of reaction by using the rate equation:
rate = k[A]ᵐ[B]ⁿ
Note that (m and n) are not coefficients of the equation, they are the reaction order of each reactant.
It is important to understand that rate law or rate order of a reaction can only be experimentally obtained.
Determining order of reaction
A simple example experimental data is given below:
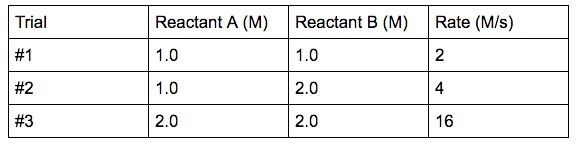
In the experiment, if we vary the concentration of one reactant at a time, we will be able to establish how the rate changes with respect to each reactant.
Reactant A is held constant, the concentration of reactant B is doubled. What is the effect on the rate? (See trial #1 & #2)
Equate the ratio
We can equate the ratio of (change in concentration) to (ratio of change in rate).
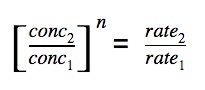
The order of reaction is simply n.
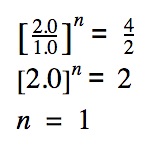
In this case, the order of reaction with respect to reactant B is 1.
The rate order is calculated for one reactant only. Now, we need to calculate the rate order with respect to the other reactant.
The order of reaction with respect to reactant A is calculated as follows:
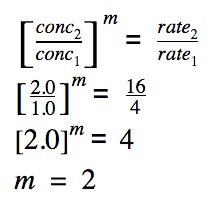
The overall order of reaction
The overall order of reaction is the sum of individual orders of reaction of the reactants.
![]()
Overall order of reaction = 3
The rate law for the reaction:
Zeroth order of reaction
The zeroth order reaction does not depend on the concentration of the reactants.

Remember that k is a constant that is dependent on temperature.
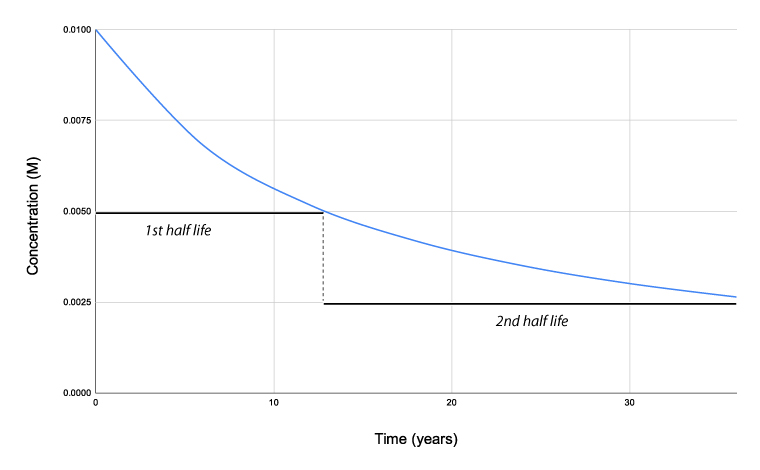
Half-life
Half life is the time it takes for half of the reactant to reach half of its concentration.

Half life for a 1st order reaction is a constant. A good example of half-life of 1st order reaction is radioactive decay. The concentration of the reactant decreases by a constant with each half–life and is independent of the concentration of the reactant. The above example shows that the concentration of the reactant decreases by half the amount every 5 years interval.
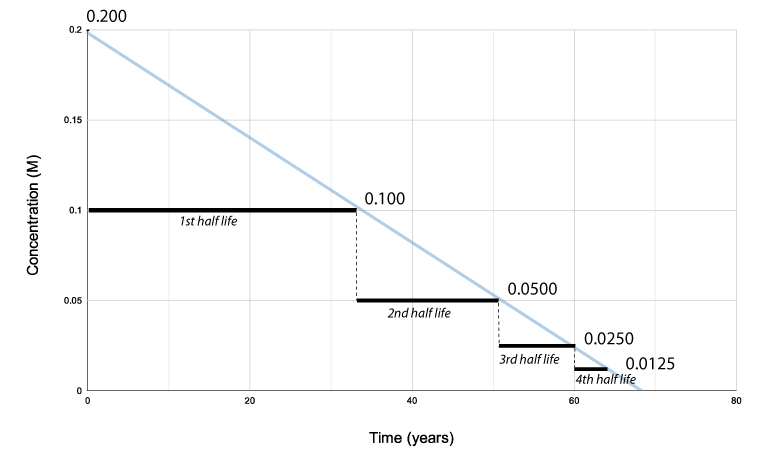
The half-life of a second-order reaction decreases as the concentration increases.
The half-life of a zeroth-order reaction decreases as the concentration decreases.
Note: Zeroth-order and second-order reactions do not have a constant half-life. Only a 1st order reaction has a constant half-life.
Summary of rate law

Zeroth order: rate does not depend on reactants
First order: rate depends on one 1st order reactant
2nd order: rate depends on two 1st order reactants or one 2nd order reactant